Converting Rx: Bowed Roller Dosage
- Published: November 30, 2005, By Timothy J. Walker, TJWalker & Assoc. Inc.
Web Lines
Last month we picked up our prescription for a bowed roller and read the instructions and warning label. But what’s in that bottle? How do we know we have the right dosage of bowed roller? Let’s combine a little diagnosis and diagramming to see how to find the proper prescription.
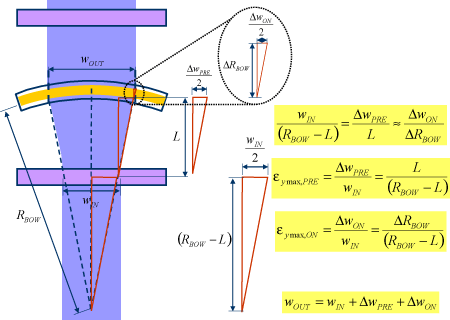
The definition of a spreader roller is that the web enters at one width, WIN, and leaves at a greater width, WOUT. In this diagram, the bow is exaggerated, making the bowed roller radius look smaller than normal relative to span length. There are three similar triangles that determine the maximum spreading of a bowed roller setup. This looks complicated at first, but there are just five critical variables: bowed roller diameter, radius of curvature, the entry span length, wrap angle, and entry span angle relative to the bow plane (the last two are not shown here).
Step 1: Calculate the effective bowed roller radius of curvature. Hold a straight edge against the inside of the bowed roller and measure the gap at the center. The length of your straight edge is the face length, F. The gap at the center is the bow, B. The bowed roller’s radius of curvature, RBOW, is equal to the face length squared divided by 8x the bow depth: RBOW=F2/8B. (This actually is a slightly simplified formula but good in typical cases where F > 10B.)
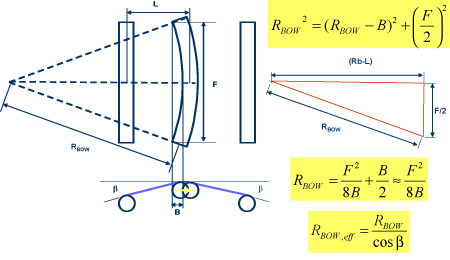
The effective radius of curvature is found by dividing RBOW by the cosine of the entry angle. For wrap angles under 20 deg, you can avoid this additional calculation. For wraps over 20 deg, this will begin to be significant.
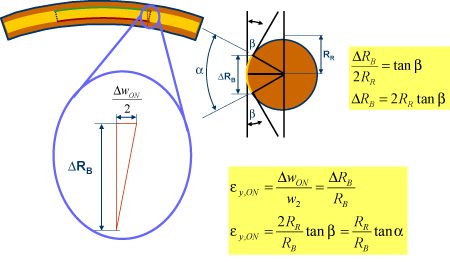
Step 2: Calculate the chord length of the bowed roller wrap. The chord length, CBOW, is the bowed roller diameter times the tangent of the entry angle. This equation assumes the default bowed roller setup where the entry and exit angles are the same relative to the bow plane.
Step 3: Calculate pre-roller and on-roller spreading. This diagram has three similar triangles. The maximum pre-roller spreading is found by comparing the first two triangles. We’ve calculated the effective RBOW and know the entry span length, L. The maximum pre-roller spreading, DWPRE/WIN, will be the L/(RBOW-L). We can use our calculated wrap angle chord length, CBOW, to find on-roller spreading. The maximum on-roller spreading, DWON/WIN, will be the CBOW/(RBOW-L). Adding the two spreading mechanisms together, the maximum combined spreading will be (L+CBOW)/(RBOW-L). Multiply this number by 100 to get percent spreading or by WIN for width change in inches. Divide the predicted width change by the number of slits to calculate the anticipated gap for spreading multiple strands.
For full web spreading, you likely won’t get to the maximum spreading for two reasons: 1) Wide webs are more beam-like and will not track in straight lines, instead shifting about two thirds of what is predicted; 2) the web will spread only as much as frictional force is available to pull the web, so spreading is often friction-limited.
How do you turn these calculations into a bowed roller prescription? For anti-wrinkle benefits and wide webs, I recommend 0.5%–1% spreading to prevent wrinkles. For strand spreading, use the calculations to get the desired gap. When you run the math, you’ll be surprised to find how easy it is to overdose on bowed roller spreading.
So, just say “no” to too much bow.

Timothy J. Walker has 20+ years of experience in web handling processes. He specializes in web handling education, process development, and production problem solving. Contact him at 651/686-5400; This email address is being protected from spambots. You need JavaScript enabled to view it.; tjwa.com.